See if you can figure out what this is (answer at the bottom)...
Mathematician says (this is nicked straight from Wiki)...
In geometry, a torus (pl. tori) is a surface of revolution generated by revolving a circle in three dimensional space about an axis coplanar with the circle. In most contexts it is assumed that the axis does not touch the circle - in this case the surface has a ring shape and is called a ring torus or simply torus if the ring shape is implicit.
A torus can be defined parametrically by:
- u,v are in the interval [0, 2π),
- R (or A) is the distance from the center of the tube to the center of the torus,
- r (or a) is the radius of the tube.
He goes further...
An implicit equation in Cartesian coordinates for a torus radially symmetric about the z- axis is
 , where
, whereAt this point the physicist steps up and says
"Give me one of those..."
 |
| a personal favorite |
 |
| A torus, bit like a doughnut if you ask me |
I argue that the language required may be so complex that it can blind people to what we are actually trying to get across.
At this time I think that sometimes, just sometimes we have to much of the mathematician in physics when what we really need is a physicist. Nice doughnut by the way.
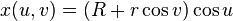



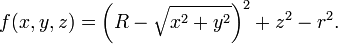




No comments:
Post a Comment